GCalc 3 Manual

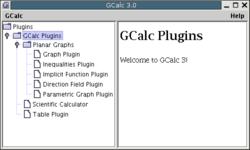
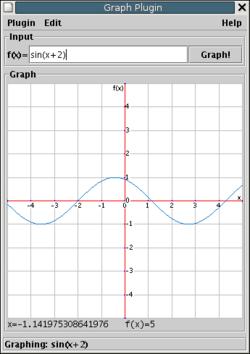
1. Quick Tutorial

Running the application

Assuming that Java 1.4 is installed on your computer, you can start GCalc 3 by clicking on the button labeled, "GCalc 3 Beta". If you do not have Java 1.4 (or higher) installed, please do that now.

First Impression

When you launch GCalc 3, the interface shown (Figure 1) will show up. On the right, there is a list of plugins that is available. As you browse through the list, the description on the right will change.

Starting a plugin

Select the plugin you want to start. Double click on its name which is displayed on the left side of the main screen. Then the plugin window should come up.

For the rest of the tutorial, let's assume that we started the Graph Plugin.

Graph Plugin

To graph a function, type in the desired function into the Input section of the window at the top of the window. Notice that you only need to put in the 'left-hand side' of the function definition since the right hand side 'f(x)=' is already provided. The following examples are valid inputs:

• -4 constant
• 2x-1 linear
• x^2+3*x+2 quadratic
• (x+2)^3-2x polynomial
• (x^2+3x+2)/(x-1) rational
• e^(-x) exponential
• sin(x+2) trigonometric
• sin(pi x)+e^x-(x+1)^3 mixed

For a more detailed description of available functions can be found in later section.

When ready, click on the Graph! button. Or more directly, press enter. If the input is well-formed, a color will be picked and the graph of the function will be shown on the Graph screen.